[10000ダウンロード済み√] 16^(x^2 y) 16x y^2)=1 219114-16^(x^2+y)+16x+y^2)=1
Hello This problem is a different question We have two different questions We have to solve them and we're gonna solve them Using raptors transforms Okay
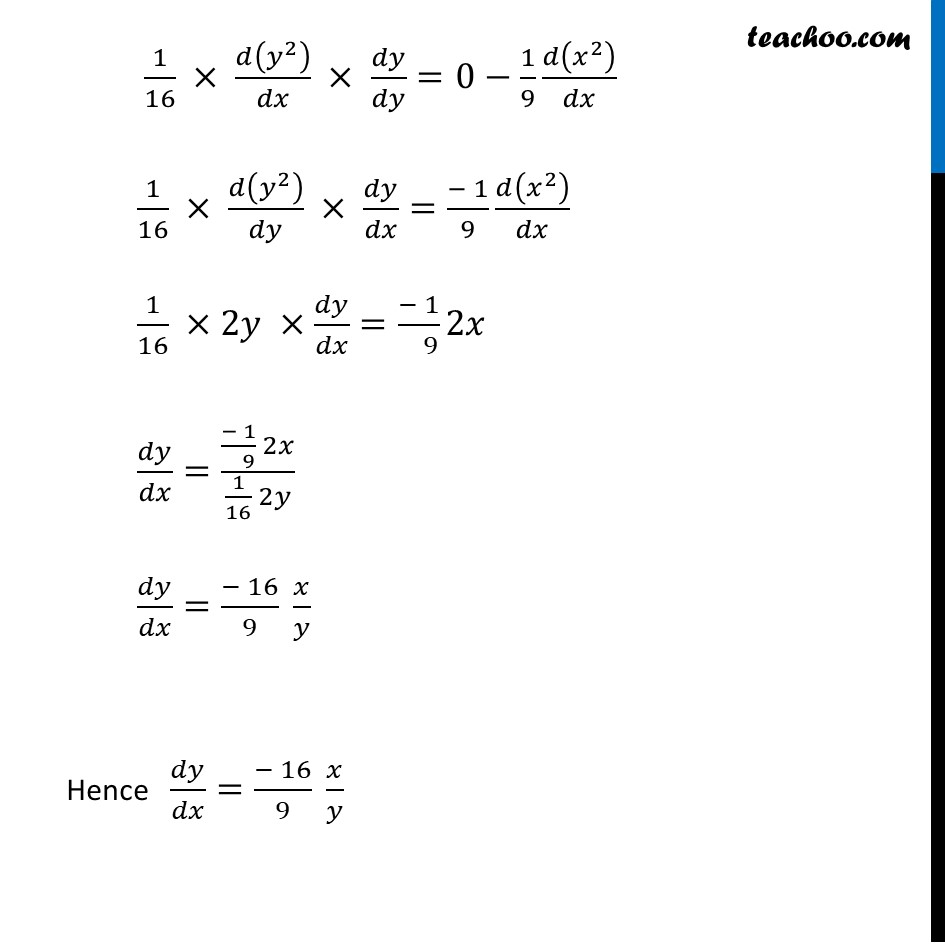
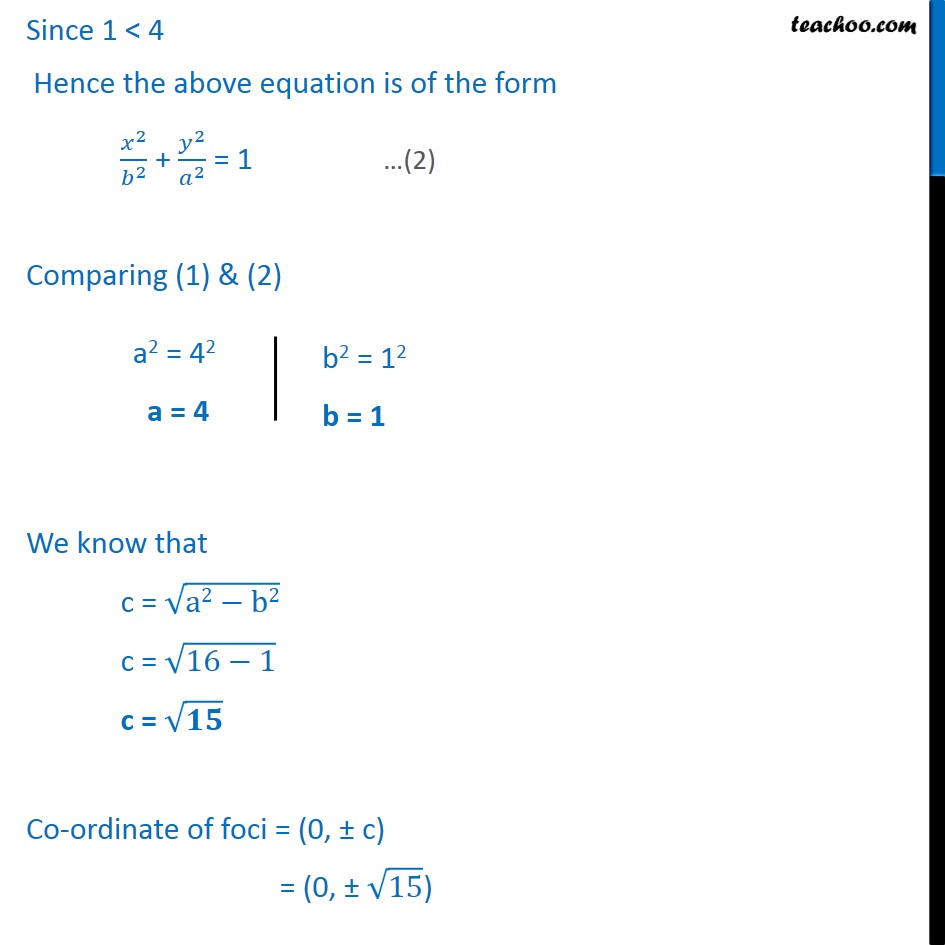
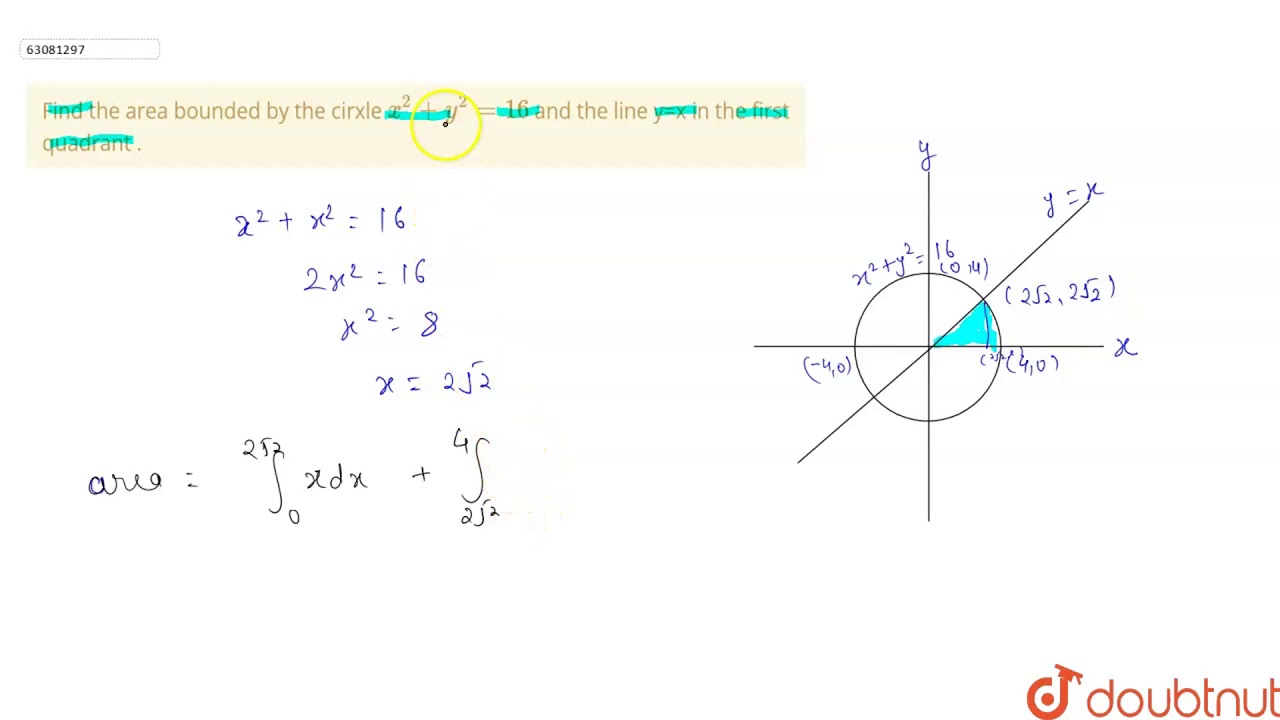
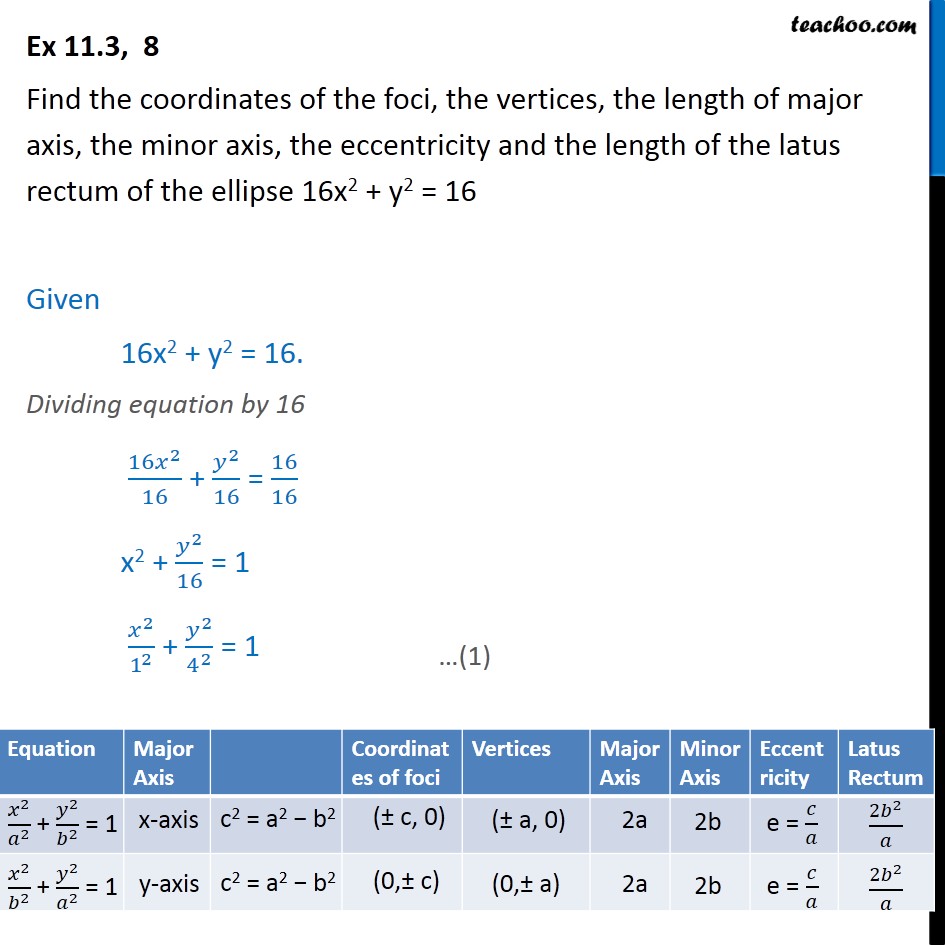
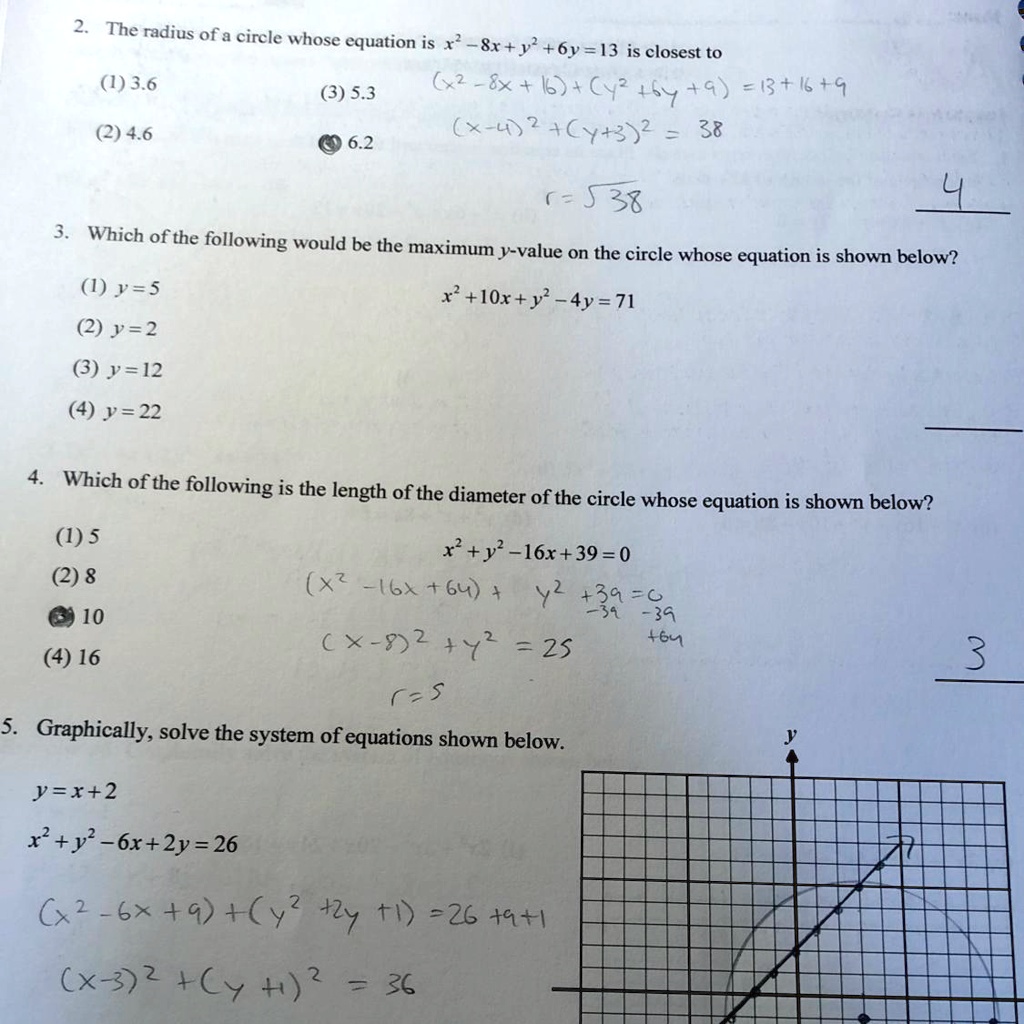
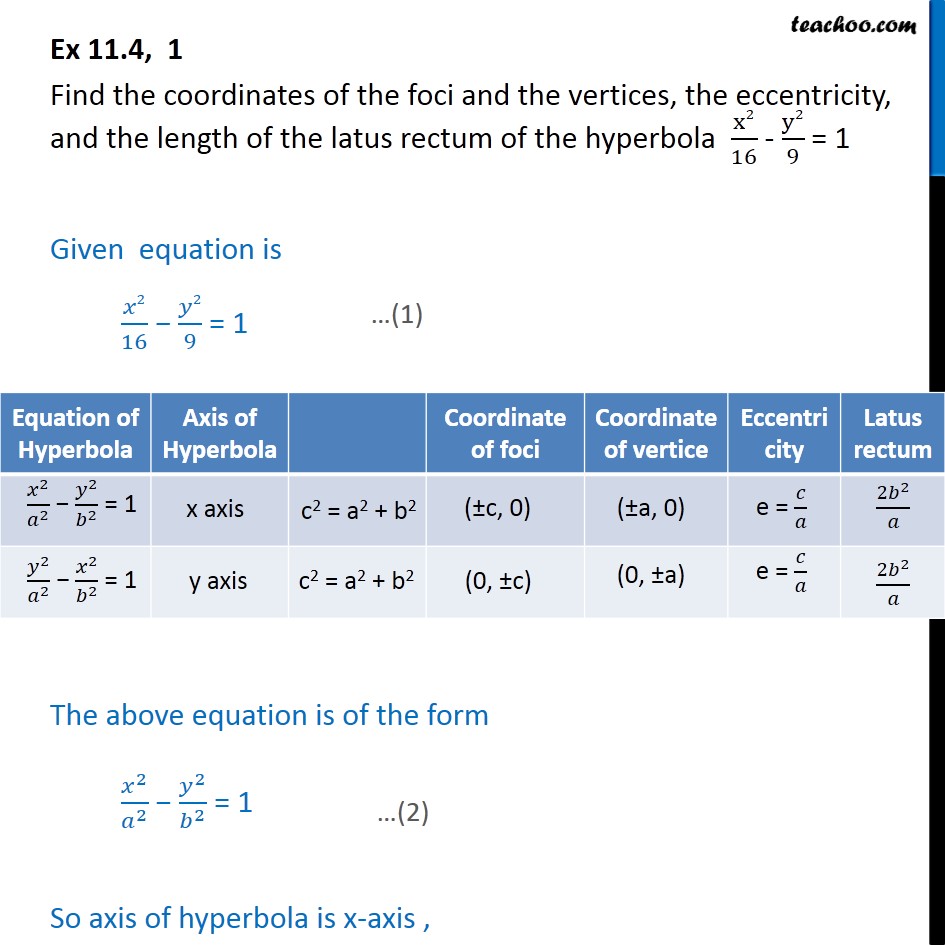
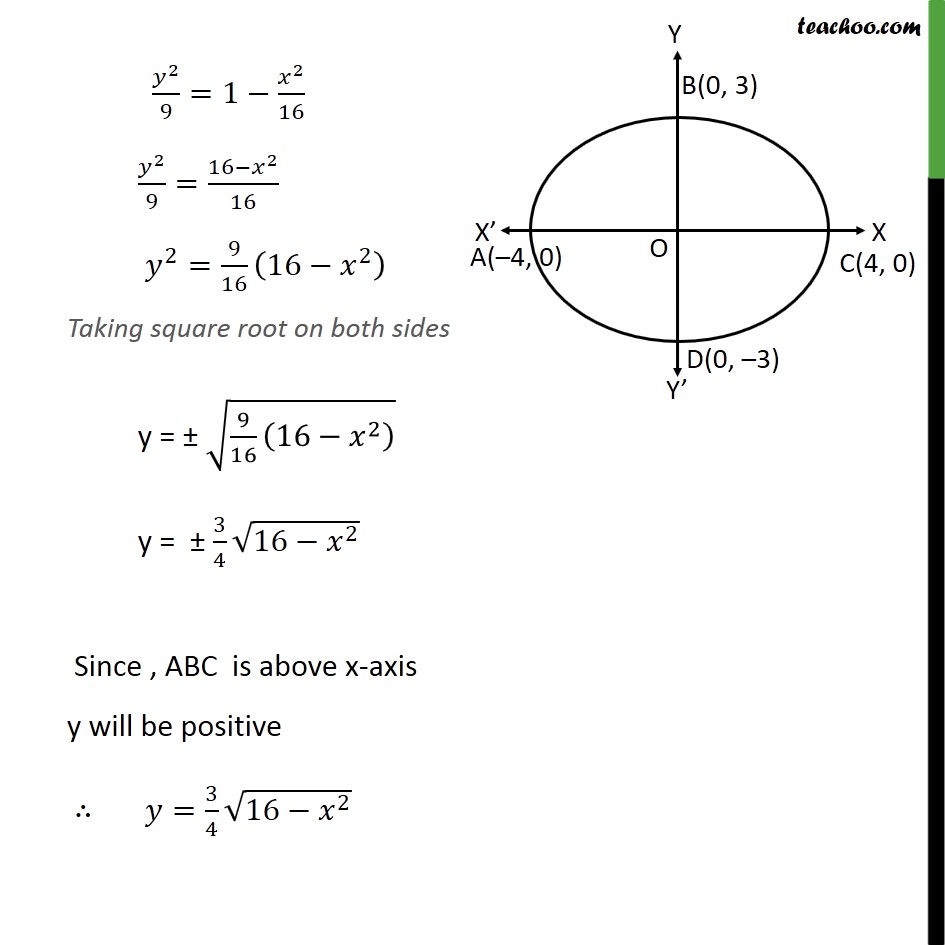
16^(x^2+y)+16x+y^2)=1- Example 14Find the coordinates of the foci and the vertices, the eccentricity, the length of the latus rectum of the hyperbolas(ii) y2 – 16x2 = 16The given equation is y2 – 16x2 = 16Divide whole equation by 16 (𝑦2−16𝑥2)/16 = 16/16 𝑦2/16 − 𝑥2/1 = 1The above equation i 16(xy) ^2x^2 solve please Get the answers you need, now!
16^(x^2+y)+16x+y^2)=1のギャラリー
各画像をクリックすると、ダウンロードまたは拡大表示できます
 |  |  |
 |  | |
「16^(x^2+y)+16x+y^2)=1」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  |  |
 |  | |
 |  |  |
「16^(x^2+y)+16x+y^2)=1」の画像ギャラリー、詳細は各画像をクリックしてください。
 | ||
 |  |  |
 |  |  |
 |  |  |
「16^(x^2+y)+16x+y^2)=1」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  | |
 |  | |
 |  |  |
「16^(x^2+y)+16x+y^2)=1」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 | ||
 | ||
 |  | |
「16^(x^2+y)+16x+y^2)=1」の画像ギャラリー、詳細は各画像をクリックしてください。
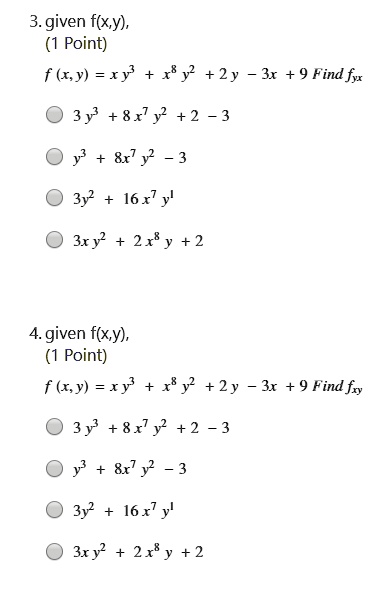 | ||
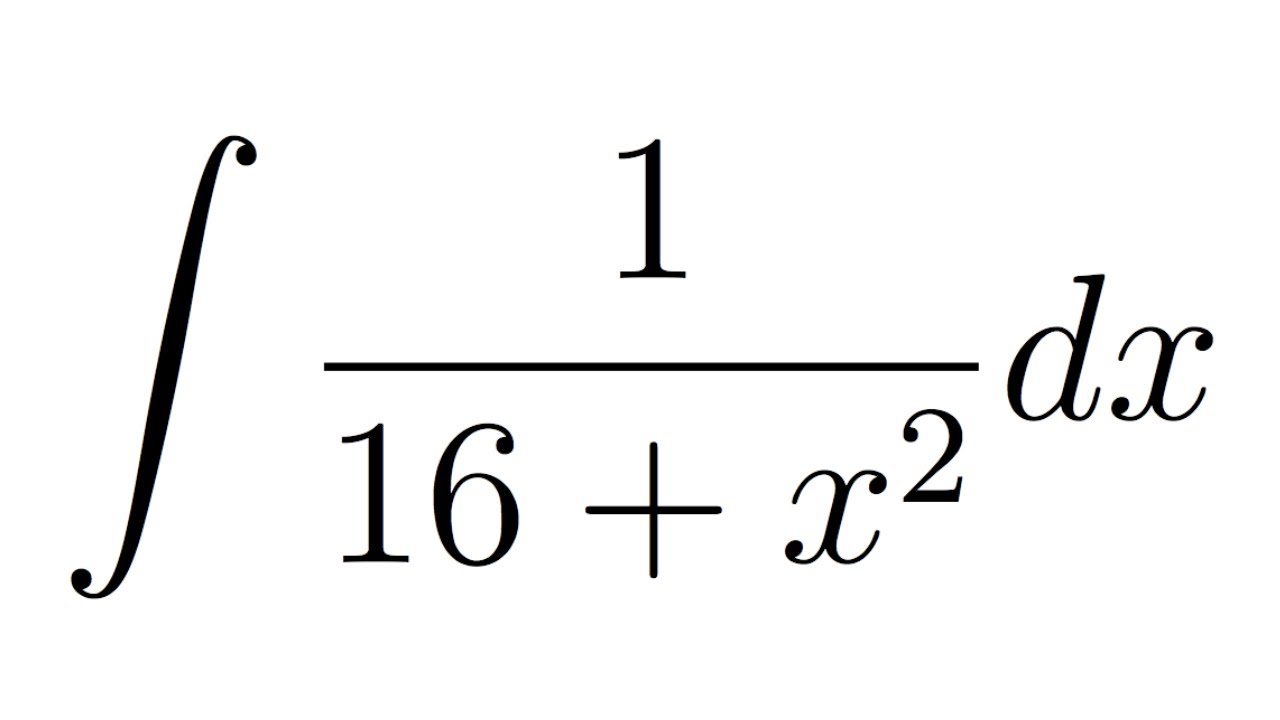 |  |  |
 | ||
 | ||
「16^(x^2+y)+16x+y^2)=1」の画像ギャラリー、詳細は各画像をクリックしてください。
 | ||
 |  | |
 |  |  |
 |  |  |
「16^(x^2+y)+16x+y^2)=1」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  | |
 |  | |
 |  | |
「16^(x^2+y)+16x+y^2)=1」の画像ギャラリー、詳細は各画像をクリックしてください。
16 / (x y ) 2 / (x – y)= 1; As you can see, this will have the same outcome as the previous, with the min/max being 16 3) y = (x16)² This is in y = (xh)² k form, but there is no k, thus the min/max k = 0 This is also true for 4) y = (x16)² Let's go back to #1 and #2 While vertex form y = (xh)² k makes finding the vertex easy, it is a lot easier to know
Incoming Term: 16^(x^2+y)+16x+y^2)=1,
コメント
コメントを投稿